20. Patterns in Nature (part four)
I’m going to wrap up my writing on patterns in nature by looking at fractals and their connection to branching patterns. In the past few months I’ve been reading an excellent series of books by Philip Ball, called Nature’s Patterns, that includes recent research, and takes a very scientific approach to the topic. One important thing I’ve realized is that we humans have a pretty strong tendency to perceive exact, symmetrical patterns in things that aren’t actually that way when looked at in measured detail. For example we talk about snowflakes having perfect symmetry, akin to the way we create paper snowflakes from cutting folded paper. However, most snowflakes examined under the microscope are not perfectly symmetrical, only close to it.
Similarly, the basic archetype of a branching pattern seems very universal across many of nature’s systems - from river networks, to trees, to human nervous systems. My initial thought was that there was some underlying principle of branching that could be extracted and expressed as a meaningful pattern in my design work. Could this underlying principle be a fractal?
Fractal Patterns
The basic definition of a fractal pattern is one that is self-similar, meaning that the pattern repeats at different scales: as you visually zoom in or out, you see the same pattern repeated in between the units of the larger pattern. A simple version of a fractal is called the Koch Snowflake, with repeated triangular shapes at various scales (see image below). The most often cited natural analogue to this pattern is a coastline, which gets more intricate the closer you zoom in on aerial photos. It brings to mind the well-known anecdote of someone trying to measure the length of a coastline, but is unable to come up with a final number after getting lost trying to measure the infinite detail of the tiniest segments of rocks and cliffs.
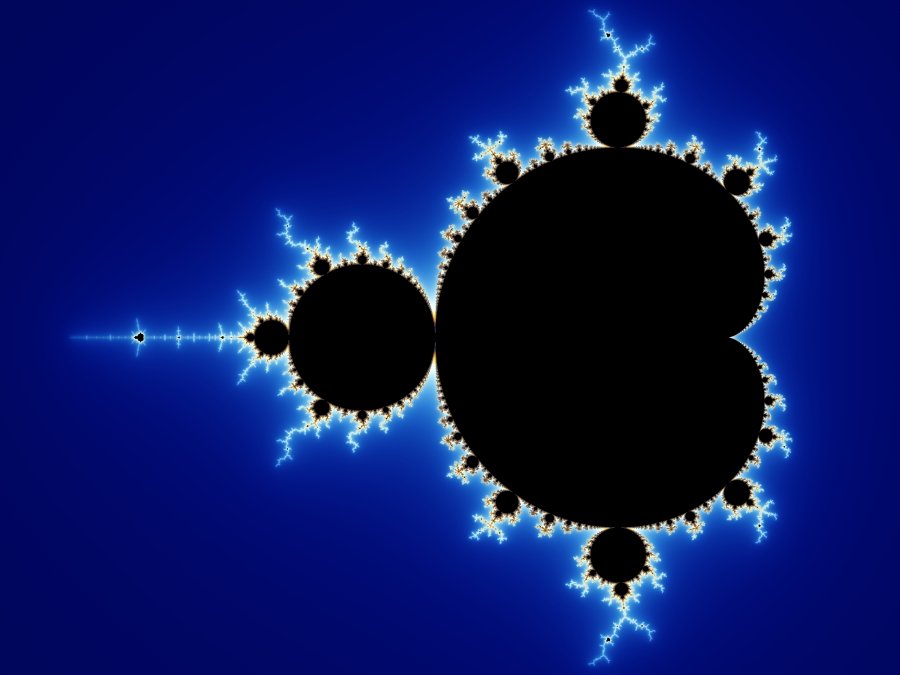
There is something almost hypnotic about fractal patterns that clicks in the human brain and seems to get a lot of people excited about them. Not too long ago, they became popular as a purely visual phenomenon, with lots of beautiful images, such as the Mandlebrot Set (image on the right), that encouraged us to see fractals everywhere. Some everyday things such as Romanesco Broccoli do have a fractal pattern, however, real objects in nature aren’t infinitely fractal, at some point it has to stop because of environmental limits and the sizes of the building blocks being used.
Fractal Branching
Many branching patterns are fractal patterns to some extent, like a tree that has a similar pattern in the largest branches and the smallest twigs. As I wrote in Part 2, branching patterns are so common in nature because of the frequent need to collect or distribute something efficiently throughout a uniform body, such as electrical impulses in a nervous system, or rain water into a river system. Philip Ball’s book mentions one researcher’s suggestion that, “it is in fact the ability of fractal networks to provide an optimal supply system to bodies of different sizes that enables living organisms to show such a huge range in body shapes and sizes, extending over 21 levels of magnification by ten from bacteria to whales.”
However, not all branching patterns are fractal, and the self-similar patterning usually only extends to two or three different scales, so I don’t think this is a key characteristic. Based on what I’ve read recently I think that there are simply too many specific environmental factors influencing any particular branch in a system to say that there is an underlying pattern archetype. Philip Ball writes, “the patterns of a river network and of a retinal nerve are both the same and utterly different. It is not enough to call them both fractal, or even to calculate a fractal dimension. To explain a river network fully, we must take into account the complicated realities of sediment transport, of changing meteorological conditions, of the specific vagaries of the underlying bedrock geology – things that have nothing to do with nerve cells.”
Inspired by Nature’s Patterns
I think that branching patterns are still a great source for design inspiration, and fractal patterns like the Koch snowflake could be used in fun ways too. I dream of fractal materials that are fabricated with self-similar intricacies, or maybe even a concrete-like material that forms fractal patterns on its own like the crystals in a snowflake, but I don’t think the materials engineers are there yet.
There are a lot of other inspiring patterns in nature that I haven’t gotten into even with a 4 part series, and surprisingly, the research on the formation of these patterns is still far from complete. My obsessive interest in the subject will keep driving me to learn more about these patterns and the possibilities of simple rules behind complex natural structures; I hope you’ve been inspired too!
References:
Nature’s Patterns, a 3 book series: Shapes, Flow, and Branches, by Philip Ball[the quotes used above come from the book Branches, pages 140 and 178 respectively]
Patterns in Nature, by Peter S. Stevens
On Growth and Form, by D’Arcy Thompson